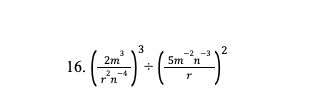@Goodness Barak thank you for this.
You got it.
Thanks for providing step by step explanation.

@goodness barak showed more workings and step by step process. I think he should take it

8m^5 ×25/r¹¹

Expanding....
[(2³ m^9) / (r^6 n-¹²)] ÷ [(5² m-⁴ n^-6) / r² ]
Moving on...... Division can now change to multiplication for the numerator and denominator to swap position...
[(8m^9) / (r^6 n-¹²)] × [(r²)/(25m-⁴n^-6)]
r² will now cancel out r² out of r^6 we now have...
[(8m^9) / (r⁴ n^-12)] × [ (1) /(25 m-⁴n^-6)]
Multiplying...
[8m^9] /[ 25r⁴ m-⁴ n^-12 n^-6)
Since n^-12 and n^-6 has the same base we'll pick one and add the powers making it n^-12+(-6) = n^-18 using the law of indices.
We now have...
[8m^9] / [25 r^4 m^-4 n^-18]
Grouping them using brackets.. We have
(8/25)(m^9 / m^-4)(1/r^4)(1/n^-18)
Using the law of indices for m^9 and m^-4 = m^9-(-4) = m^13
(8/25)(m^13)(1/r^4)(1/n^-18)
Opening back the brackets
[8m^13] / [25r^4 n^-18]
This can be the simplified form but it can also be written as
[8m^13 r^-4 n^18] / 25.

8m^5/25r³n–²