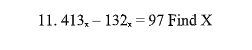@Emmanuel Ocheme he got it.

x=2

@oluwasegun solaja does that mean that he is wrong or what?

@Emmanuel Ocheme thank you for this explanatpry solution. You got it.
You are right but it is only applicable if you are to use the output of the equation somewhere else.
Negative numbers are numbers as well.

Kudos.

Change the parameters on LHS to decimal:
413x = 4 × x² + 1 × x¹ + 3×x⁰
= 4x²+x+3
132x = 1×x² + 3×x¹ + 2×x⁰
= x²+3x+2
Bring both expression together:
=> 4x²+x+3 - (x²+3x+2) = 97
4x²+x+3-x²-3x-2 = 97
3x² -2x+1 = 97
Subtract 97 from both sides:
3x²-2x+1-97 = 97-97
3x²-2x+1-97 = 0
3x²-2x-96 =0
Using formula method to solve the quadratic equation:
x = (-b +- √b²-4ac) / 2a
Where a=3, b=-2 and c=-96
x = (-(-2) +- √(-2)²-4×3×-96) / 2×3
x = (2 +- √4+1152) / 6
x = (2 +-.√1156) / 6
x = (2 +- 34) / 6
Therefore x is either;
(2+34) / 6 or (2-34) / 6
x = 36/6 or x = -32/6
x=6 or x=-16/3
When finding unknown numbers, unless it is specified that you should find the negative number, you should always use the positive number as your final answer.
So X= 6.